An article from Norwegian SciTech News at NTNU

A new solution to an age-old equation can improve ship efficiency
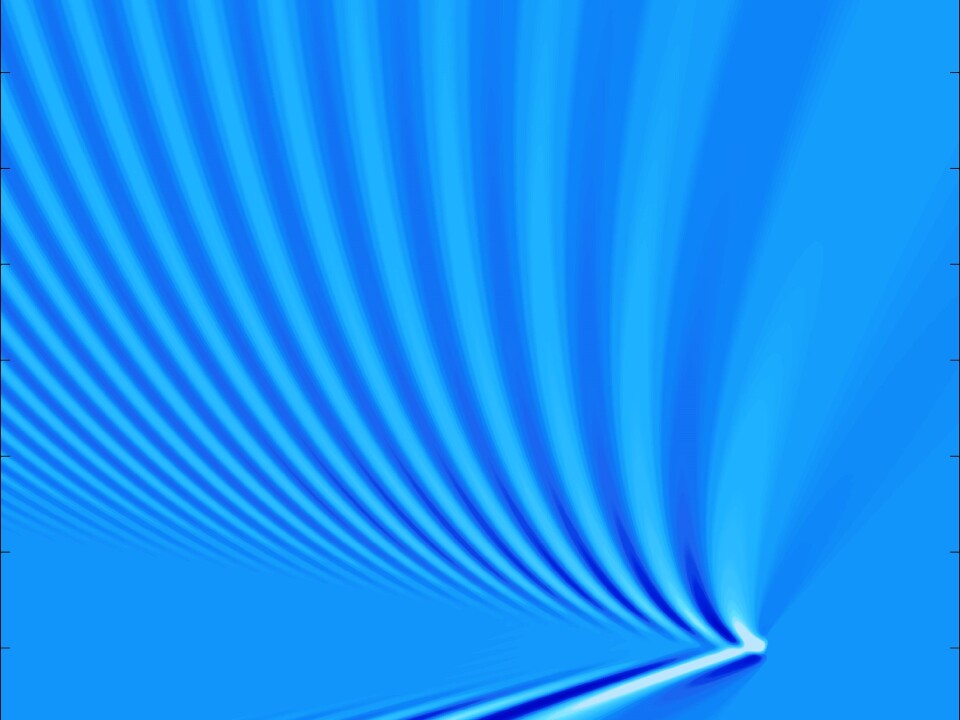
It’s been well known for 127 years – the V-shaped wake pattern behind a ship moving in a straight line always has the same central angle. Now a Norwegian has discovered that in certain situations, a boat’s wake can actually be found in front of the boat.
Denne artikkelen er over ti år gammel og kan inneholde utdatert informasjon.
The Kelvin angle of 19 degrees, 28 arc minutes is famous among mathematicians and physicists because it is simple and universal.
Lord Kelvin’s theory states that wake patterns will always have the same central angle, no matter if it is a ship, a swan or a kayak moving through the water. This should be universal, as long as the water is deeper than a boat length or two. It’s been this way since 1887.
But armed with a pen and paper, Simen Ådnøy Ellingsen, an associate professor at NTNU’s Department of Energy and Process Engineering, recently discovered that it does not always work that way after all.
The angle of a wake pattern may actually vary, and Ellingsen has discovered why.
“I think the problem was assumed to be difficult because standard wave theory methods cannot be used for the expansion I’ve looked at. But for me this was a totally new field, and nobody told me it was supposed to be hard,” Ellingsen says.
A wake in front of the boat?
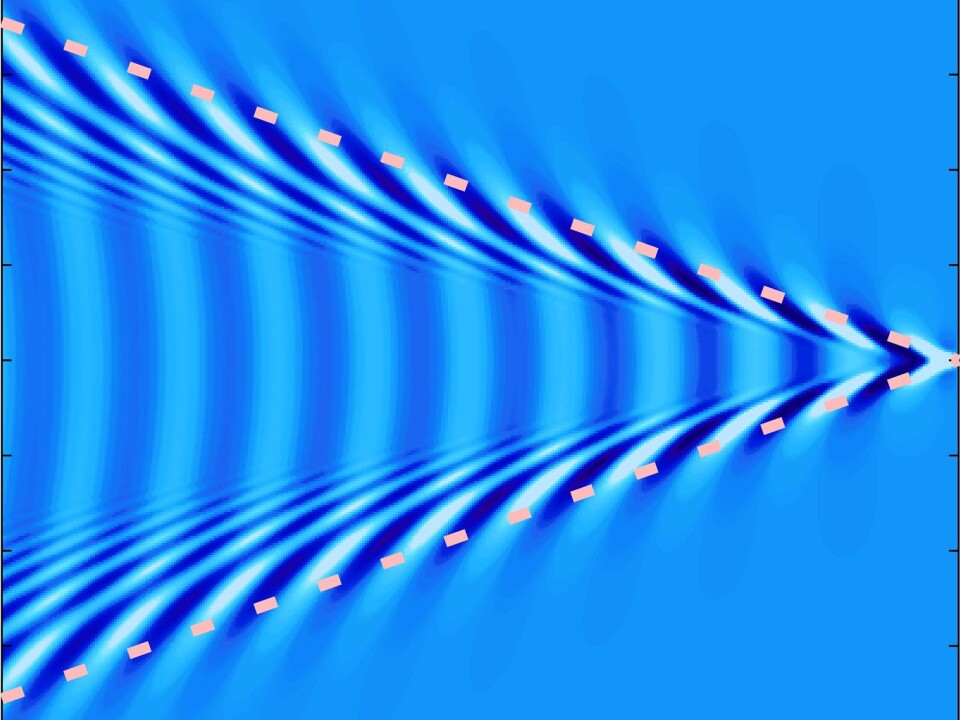
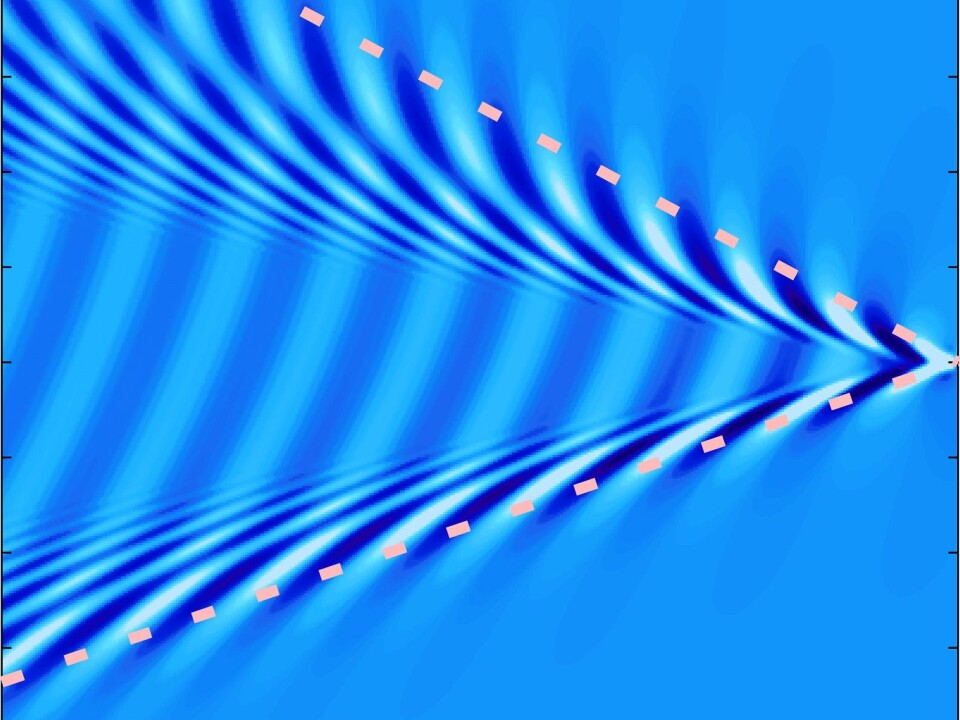
He views his results as an expansion on Kelvin’s theory. As long as the water under the surface is still, Kelvin’s angle is correct. But as soon as there is turbulence, water moving at different speeds in different layers, the angle changes. Sometimes by a lot.
The angle of a wake pattern does not have to be narrow any more. In theory, and maybe in practice as well, the angle can become wide enough that the wake could travel out in front of a boat on one side.
“It’s really cool,” Ellingsen says.
For the sake of clarity, the angle in question is the one between the wave front and the direction of the boat’s movement. The complete central angle, which is the V-shaped wake you see, is twice as large, just under 39 degrees. But mathematically, the former angle is easier to work with.
May help reduce fuel use
Does Ellingsen’s work have a practical application? Yes, actually.
“About half of the fuel burned by a ship goes to making these wave patterns,” Ellingsen points out.
This wave energy is basically completely wasted, but Ellingsen’s work may contribute to reducing fuel use, thus saving money and the environment.
To reduce consumption, we need to understand how waves behave. At river inlets, where undercurrents form, or when wind sets the top layers of water in motion, the waves and the central angle of a wake pattern may be completely different. But calculating fuel use for these circumstances is not necessarily an issue any more

“With my theory, you can take a boat of any size and shape moving at any speed, with a current under the water’s surface, and calculate the resistance caused by the wake,” he says.
Most boats travel through relatively still waters, although this is not necessarily the case for river boats, for example.
Solved on a piece of paper
Ellingsen made his discovery using pen and paper. Although he did use a computer program to simulate boat wakes under different conditions, most of the work was done the old-fashioned way.
“I’d say this really is a typical pen and paper problem,” he said.
He is a little surprised that this expansion on Kelvin’s classic theory hasn’t been found before.
“You would think that somebody would have done this before 1930, but that isn’t the way it is,” he said.
Fewer and fewer people actually use the “old” method for finding the answers to difficult problems. He thinks that the widespread use of computers may actually be one of the reasons that nobody has discovered this before.
“There aren’t that many people left who use a pen and paper like me,” Ellingsen says.
Fun to include
Ellingsen is not actually a specialist in this area at all. He was looking for exercises and subjects that he could include in a course in advanced fluid mechanics for PhD students.
“I thought it would be fun to include some wave theory,” Ellingsen says.
He came across the Kelvin angle theory, and decided it would be good to let the students explore it. Another part of the curriculum was about how underwater currents can affect waves in two dimensions.
“It hit me that maybe these two problems could be combined,” he says.
Two months later, the whole thing was solved. Kelvin’s theory was expanded to include cases with subsurface currents. Another two months, and Ellingsen’s work was on the cover of the prestigious Journal of Fluid Mechanics.